MPAC 2 – Connections

Posted on by
Reply

steve-jobs-quote-about-creativity-1

-Steve Jobs

Continuing the series on the Mathematical Practices for AP Calculus (MPACs) today we look at MPAC 2.

MPAC 2: Connecting concepts

Students can:

a. relate the concept of a limit to all aspects of calculus;

b. use the connection between concepts (e.g., rate of change and accumulation) or processes (e.g., differentiation and its inverse process, antidifferentiation) to solve problems;

c. connect concepts to their visual representations with and without technology; and

d. identify a common underlying structure in problems involving different contextual situations.

— AP® Calculus AB and AP® Calculus BC Course and Exam Description Effective Fall 2016, The College Board, New York © 2016. Full text is here.

While “limit” seems to disappear shortly after the definition of derivative is past and reappears briefly with the definition of the definite integral, in fact all of the calculus depends on limits. Limit seems to be used for other things – continuity, end behavior, asymptotes – but really limit is what makes all of the calculus work and provides the firm foundation for derivatives and integrals and therefore is always in the background of everything calculus. Students need to be made aware of this.

Connecting the concepts in calculus and in previous work in mathematics, seeing the same ideas in different contexts, and using one concept in different ways to solve different type of problems is what makes mathematics in general and the calculus in particular so universal in its application and effectiveness. The ideas in mathematics relate to each other; they are not separate items.

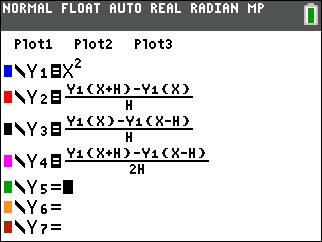
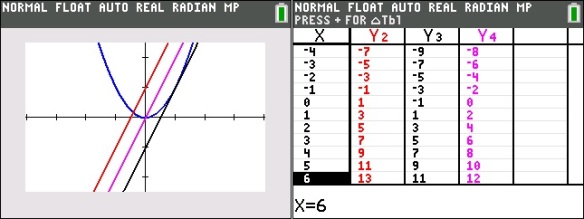
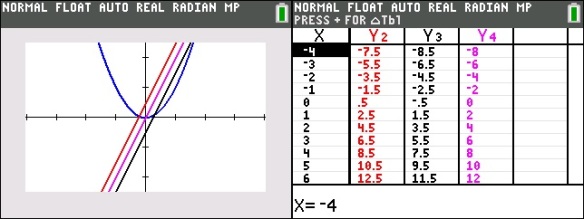
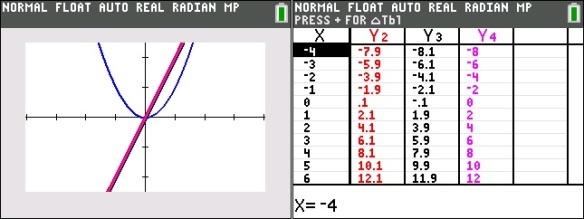
The “Rule of Four” helps students see and understand these connections; technology makes the Rule of Four easy to apply in multiple situations.

How/where can you make sure students use these ideas in your classes.

All the way through the teaching and learning of mathematics these connections exist. Teachers need not only to be aware of them but be sure to point them out to students. Whenever there is an equation, discuss what it means in the context of the problem, see what its graph tells you, and, when a new use comes up, relate it to the previous applications. This is not intended as a way to address different learning styles. The Rule of Four approach is for all students – some will see the idea better on way or the other, but all students will benefit from seeing the connections and the various approaches.

The MPACs overlap with each other. Building notational fluency (MPAC 5), attending to the proper implication of algebraic and computational processes (MPAC 3), connecting multiple representations (The Rule of Four, MPAC 4), proper reasoning (MPAC 1), and communicating the ideas (MPAC 6) all lead to connecting the concepts.

steve-jobs-quote-about-creativity-2

When AP exam questions are written the writers reference them to the LOs, EKs and MPACs. The released 2016 Practice Exam given out at summer institutes this summer is in the new format and contains very detailed solutions for both the multiple-choice and free-response questions that include these references. (This version is not available online as far as I know.) About 40% of the multiple-choice and all six free-response questions on both AB and BC exam reference MPAC 2.

Here are some previous posts om these topics

Limits

Examples of connecting the concepts of graphing functions and linear motion problems

PLEASE NOTE: I have no control over the advertising that appears on this blog. It is provided by WordPress and I would have to pay a great deal to not have advertising. I do not endorse anything advertised here. I noticed that ads for one of the presidential candidates occasionally appears; I certainly do not endorse him.

.

MPAC 1 Reasoning

Posted on by
Reply

My series on calculator/technology use will continue during the year. Meanwhile, today I am starting a short series about Mathematical Practices for AP Calculus or MPACs as they are called.

Earlier this year I did some work verifying the alignment of several textbooks to the Essential Knowledge (EK), Learning Objectives (LO), and MPACs of the new Course and Exam Description for AP Calculus. The publishers provided a reference (page or exercise number) for each LO and EK in their books and a separate reference for each MPAC. The books I looked at all hit the LOs and EKs pretty well at over 95%. But the MPACs, not so much.

The MPACs are intended to get students thinking and working like mathematicians. The more I look at them, the more I think they can do that.

Each MPAC starts with the statement “Students should ….” It’s easy for authors to do a good job explaining the calculus in the LOs and EKs; that’s what authors do. The writers do what’s listed in the MPACs because they are good mathematical practices and the authors are good mathematicians, but the authors do not often point out what they are doing in this regard.

The MPACs are for students to do. Textbooks need to provide opportunities for students to do the them. This pretty much has to be in the exercises. Some of the exercises provide the opportunity to do some of the things listed in the MPACs, but this is often more accidental than intended.

Little or no opportunity is intentionally provided to learn and practice the MPACs. It is up to the teachers to provide these opportunities.

While they are called Mathematical Practices for AP Calculus, in fact they really apply to all of mathematics. The calculus examples in the MPACs may easily be changed to apply to mathematics teaching and learning earlier in the curriculum. Properly applied they should have an impact on the entire curriculum. If the goal is to help students learn to think and work like mathematicians, then starting in AP Calculus is way too late.

I will use this and the next few post to discuss the MPACs in detail and provide some suggestions as to where and how teachers can help their students to think and work like mathematicians.

MPAC 1: Reasoning with definitions and theorems

Students can:

a. use definitions and theorems to build arguments, to justify conclusions or answers, and to prove results;

b. confirm that hypotheses have been satisfied in order to apply the conclusion of a theorem;

c. apply definitions and theorems in the process of solving a problem;

d. interpret quantifiers in definitions and theorems (e.g., “for all,” “there exists”);

e. develop conjectures based on exploration with technology; and

f. produce examples and counterexamples to clarify understanding of definitions, to investigate whether converses of theorems are true or false, or to test conjectures.

— AP® Calculus AB and AP® Calculus BC Course and Exam Description Effective Fall 2016, The College Board, New York © 2016. Full text is here.

While the word logic does not appear here, these six items (with the possible logic-spockexception of e) are the tools of logic and the basis of mathematical reasoning. The word prove has appeared very rarely on the AP Calculus exams, students have been asked to justify their answers, apply a definition or theorem to a particular function, and show that they know what ideas can be used in a situation and show that they can use them. Conjecturing, producing examples and counterexamples are the basis of mathematical reasoning.

Some suggestions about how and where you can make sure students work with these ideas in your classes.

These items reflect the structure of mathematics. None of the points are specific to the calculus; they can and should be used and developed in all the classes leading up to calculus. Definitions and theorems come into students’ mathematical education before the first year of algebra. The form and structure of axioms, definitions, and theorems, in addition to their meaning, should be made clear to students. So this is something that should start long before calculus and be included every year.

One way you can help students learn how the items in MPAC 1 work is to use True or False (TF) questions; better yet are Always, Sometimes or Never (ASN) questions. These are similar to TF questions except that the students have a middle choice. These questions are an excellent place to hone ones’ skills using the fine points of theorems and definitions. With either TF or ASN questions students should not just answer with a word, but rather be required to explain how they know their answer is correct. They can do this by citing some theorem or definition or producing an example or counterexample. Students can also be asked to discuss, defend, and compare and contrast their answers with other students.

We all know that AP exam questions often require students to “Justify your answer” or “Explain your reasoning.” Here, too, is a good place to practice with the skills of MPAC 1, since justifications and explanations are based on the theorems and definitions

When AP exam questions are written the writers reference them to the LOs, EKs and MPACs. The released 2016 Practice Exam that is in the new format contains very detailed solutions for both the multiple-choice and free-response questions that include these references. About 1/3 of the multiple-choice and all six free-response questions on both AB and BC exam reference MPAC 1.

Here are some previous posts on these subjects:

I have discussed definitions and theorems in previous posts. Here are links to some of them:

PLEASE NOTE: I have no control over the advertising that appears on this blog. It is provided by WordPress and I would have to pay a great deal to not have advertising. I do not endorse anything advertised here. I noticed that ads for one of the presidential candidates occasionally appears; I certainly do not endorse him.

Comparing the Graph of a Function and its Derivative

Posted on by
4

The fourth in the Graphing Calculator / Technology series

Comparing the graph of a function and its derivative is instructive and necessary in beginning calculus. Today I will show you how you can do this first with Desmos a free online graphing program and then on a graphing calculator. Desmos does this a lot better than graphing calculators, because of the easy use of sliders. CAS calculators also have sliders but they are not as easy to use as Desmos.

Let’s get started. Instead of presenting you with a completed Desmos graph, I will show you how to make you own. One of the things I have found over the years is that it takes some mathematical knowledge to make good demonstration graph and that in itself if useful and instructive. Hopefully, you and your students will soon be able to make your own to show exactly what you want.

Open Desmos and sign into your account; if you don’t have one then register – its free and you can keep your results and even share them with others.

In the first entry line on the left, enter the equation of  the function whose graph you want to explore. Call it f(x); that is enter f(x) = your function. Later you will be able to change this to other functions and investigate them, without changing anything else.

On the second line enter the symmetric difference quotient as

\displaystyle s\left( x \right)=\frac{f\left( x+0.001 \right)-f\left( x-0.001 \right)}{2\left( 0.001 \right)}

Instead of a variable h, as we did in our last post in this series, enter 0.001. This will graph the derivative without having to calculate the derivative. Of course, you could enter the derivative here if your class has learned how to calculate derivatives. If so, you will have to change this line each time you change the function.

In order to closely compare the function and its derivative, on the next line enter the equation of a vertical segment from a point on the function (a, f(a)) to a point on the derivative (a, s(a)). Desmos does not have a segment operation, but here is how you graph a segment. In general, a segment from (a, b) to (c, d) is entered as the parametric/vector function

\left( a\cdot t+c\cdot \left( 1-t \right),b\cdot t+d\cdot \left( 1-t \right) \right),\ 0\le t\le 1

The a, b, c, and d may be numbers or functions. Since our segment is vertical the first coordinate will have a = c and will reduce to a. Here’s what to enter on the third line:

\left( a,f\left( a \right)\cdot t+s\left( a \right)\cdot \left( 1-t \right) \right)

(Notice that there is no x in this expression; t is the variable. Also, the f(a) and s(a) may be interchanged.)

When you push enter, you will be prompted to add a slider for a: click to add the slider. A line will appear under the expression which will allow you to set the domain for t: click the endpoints and enter 0 on the left and 1 on the right, if necessary.

That’s it. You’re done. Use the slider to move around the graphs.

Using the graphs

Discuss with your class, or better yet divide them into groups and let them discuss, what they see. Since at this point they are probably new to this provide some hints such as “What happens on the graph of  f when s is 0?” or “What is true on s when f is increasing?” or “What happens to the function at the extreme values of the derivative?” Prompt the students to look for increasing and decreasing, concavity, points of inflection, and extreme values. All the usual stuff. Work from the function to the derivative and from the derivative to the function.

Have your students formulate their results as (tentative) theorems.  You actually want them to make some mistakes here, so you can help them improve their thinking and wording. For example, one result might be:  If the function is increasing, then the derivative is positive. By changing the first function to an example like f(x) = x3 or f(x) = x + sin (x). Help them see that non-negative might be a better choice.

You might try giving different groups different functions and let them compare and contrast their results.

This is very much in line with MPACs 1, 2, 4, and 6.

You can do the same kind of thing with graphing calculators. That is, you can graph the function and its derivative or a difference quotient. The difference is that graphing calculators do not have sliders.

Extra feature: Desmos will graph a point if you enter the coordinates just like you write them: (a, b). The coordinates may be numbers or functions or a combination of both. Try adding two points to your graph one at each the end of the segment between the graphs that will move with the same slider.

f(x) = x + 2sin(x) and its derivative.

f(x) = x + 2sin(x) and its derivative.

 

 

 

 

 

 

 

 

Seeing Difference Quotients

Posted on by
Reply

Third in the graphing calculator series. 

In working up to the definition of the derivative you probably mention difference quotients. They are

The forward difference quotient (FDQ): \displaystyle \frac{f\left( x+h \right)-f\left( x \right)}{h}

The backwards difference quotient (BDQ): \displaystyle \frac{f\left( x \right)-f\left( x-h \right)}{h}, and

The symmetric difference quotient (SDQ): \displaystyle \frac{f\left( x+h \right)-f\left( x-h \right)}{2h}

Each of these is the slope of a (different) secant line and the limit of each as, if it exists, is the same and is the derivative of the function f at the point (x, f(x)). (We will assume h > 0 although this is not really necessary; if h < 0 the FDQ becomes the BDQ and vice versa.)

To see how this works you can graph a function and the three difference quotients on a graphing calculator. Here is how. Enter the function as the first function on your calculator and the difference quotients with it. Each of the difference quotients is defined in terms of Y1; this allows us to investigate the difference quotients of different functions by changing only Y1.

DQ 1

Now, on the home screen assign a value to h by typing [1] [STO] [alpha] [h]

Graph the result in a square window.

The look at the table screen. (Y1 has been turned off). Can you express Y2, Y3, and Y4 in term of x?

DQ 2

Then change h by storing a different value, say ½, to h and graph again. Then look at the table screen again. Can you express Y2, Y3, and Y4 in term of x?

DQ 3

Then graph again with h = -0.1

DQ 4

As you can see as h gets smaller (h 0), the three difference quotients are FDQ: 2x + h, the BDQ is 2x – h, and the SDQ is 2x. They converge to the same thing. The limit of each difference quotient as h approaches zero is twice the x coordinate of the point. If you’re not sure try a smaller value of h.

The function to which each of these converge is called the derivative of the original function (Y1). In the example the derivative of x2 is 2x.

Now try another function say, Y1 = sin(x) and repeat the graphs and tables above. The tables will probably not be of much help, since the pattern is not familiar. The graph shows the function (dark blue) and only the SDQ (light blue), h = 0.1 Can you guess what the derivative might be?

DQ 5

I f you guessed cos(x) you are correct. The table shows the SDQ values as Y4, and the values of cos(x) as Y5. Pretty close! If you want to get closer try h = 0.001.

DQ 6

If you have a CAS calculators such as the TI-Nspire or the HP PRIME you can do this activity with sliders. Also you may try this with DESMOS. Click here or on the graph below. Some interesting functions to start with are cos(x) and | x |.

And by the way, the SDQ is what most graphing calculators use to calculate the derivative. It is called nDeriv on TI calculators.

Tangent Lines

Posted on by
Reply

Second in the Graphing Calculator/Technology series

This graphing calculator activity is a way to introduce the idea if the slope of the tangent line as the limit of the slope of a secant line. In it, students will write the equation of a secant line through two very close points. They will then compare their results in several ways.

Begin by having the students graph a very simple curve such as y = x2 in the standard window of their calculator. Then TRACE to a point. Students will go to different points, some to the left and some to the right of the origin. ZOOM IN several times on this point until their graph appears linear (discuss local linearity here). To be sure they are on the graph push TRACE again. The coordinates of their point will be at the bottom of the screen; call this point (a, b). Return to the home screen and store the values to A and B (click here for instructions on storing and recalling numbers).

Return to the graph and push TRACE again to be sure the cursor is on the graph. Move the TRACE cursor one or two pixels away from the first point in either direction. This new point is (c, d). Return to the home screen and store the coordinates to C and D.

calc 2.1

Enter the equation of the line through the two points on the equation entry screen in terms of A, B, C, and D. Zoom Out several times until you have returned to the original window..

calc 2.4

Exploration 1: Have students compare and contrast their graphs with several other students and discuss their observations. (Expected observations: the lines appear tangent at each students’ original point)

Exploration 2: Ask student to compute the slope of the line through their points, again using A, B, C, and D. Collect each student’s x-coordinate, A, and their slope and enter them in list is your calculator so that they can be projected.

calc 2.2

Study the two lists and discuss the relation is any. (Expected observations: the slope is twice the x-coordinate.) Can you write an equation of these pairs? (Expected result: y = 2x)

Finally, plot the points on the calculator using a square window. Do the points seem to lie on the line y =2x?

calc 2.3

Extensions:

Try the same activity with other functions such as y = (1/3)x3, y = x3, or y = x4. Anything more difficult will still result in a tangent line, but the numerical relationship between x and the slope will probably be too difficult to see. You may also consider y = sin(x) or y = cos(x). Again, the numerical work in Exploration 2, will be too difficult to see, but on graphing the points the result may be obvious. For y = sin(x), return to the list and add a column with the cosines of the x-values. Compare these with the slopes.

Graphing Calculator Use

Posted on by
Reply

First in a series. 

I am going to (try to) write a series of posts this fall on graphing calculator use in for calculus. Graphing calculators became generally available around 1989 and were made a requirement for use on the AP calculus exams in 1995. The hope was that they would encourage the use of technology generally in all math classes, and to an extent this has happened. In the coming posts I hope to show how graphing calculators can be used beyond the four skills required for the AP exams to help students understand what’s going on.

I will occasionally work with CAS calculators, but the main focus will be the basic non-CAS graphing calculators. All the suggestions will work of CAS calculators, of course. Also, I will occasionally make use of Desmos a free online graphing utility that students can easily access on their computer, tablet or smart phone.

Today I will discuss the four things students should be able to do, and in fact are required to do, on the AP exams. I will also show how to store and recall numbers. This last skill, while not required, is very useful. I have found that some teachers, and therefore their students, are unaware of this common feature of graphing calculators.

Calculators and other technology should be available from at least Algebra 1 on. So by the time students get to calculus calculator use (except for the calculus specific operations) should all be second nature to them.

So let’s get going. Here are the four required skills and some brief comments on each.

  1. Graph a function in a suitable window. The exam questions do not say “use a calculator”, so students are expected to know when seeing a graph will help them. The viewing window is not usually specified either so students should be familiar with setting the viewing window. If the domain is given in the question, then that’s what the students should use. If no domain is given, then use a range that includes the answer choices.
  2. Solve an equation numerically. Students may use any built-in feature to do this. The home screen “solver”, a routine called “Poly” or “Poly solve” are all allowed. Probably the most useful is to graph both sides of the equation and then use the graph operation called “intersect” to find the points of intersection one at a time. Another approach is to set the equation equal to 0, graph that expression and use the “roots” or “zeros” operations to solve the equation.
  3. Find the value of the derivative of a function at a given point. There is a built-in template for this.
  4. Find the value of a definite integral. There is a built-in template for this also.

Store-and-Recall

I will demonstrate the store-and-recall idea with an example that will also use skill 1, 2, and 4 from the list above.

Example: Find the area between the graph of f(x) = ln(x) and g(x) = x – 2, using (1) vertical rectangles, and (2) using horizontal rectangles.

For either method we need the coordinates of the points of intersection of the graphs. So, begin by graphing the functions and adjusting the window so the important points are visible.

calc 1Next use the intersection operation to find the first point of intersection.

calc 2

Different calculators will do this slightly differently. The coordinates of the points of intersection are shown at the bottom of the screen. Think of this point as (a, b). The coordinates need to be stored for use later. To do this return to the home screen and type

[x] [STO], [alpha] [A]    and    [alpha] [y] [STO] [alpha] [B]

If your graph screen shows different names, such as xc and yc, then type that befroe the [STO] key. (The store [STO] key may look like an arrow pointing right.)

calc 3

Return to the graph screen and find the coordinates of the second point of intersection; think of this as (c, d). Return to the home screen and store the coordinates as C and D. They are c = 3.146193221 and d = 1.146193221.

To find the area use the definite integral template and enter the information needed. For the vertical rectangles you may use either the function or Y1 and Y2 that you entered to graph.

calc 4

Notice that the upper and lower limits of integration are A and C. The calculator uses the values you stored in these locations. You can use the variables in any kind of computation.

Here is the horizontal rectangle computation using B and D as limits. The functions solved for y are x = y + 2 on the right and x = ey on the left. (The y’s have been changed to x’s since the template only allows x as a variable.)

calc 5

Notice that so far, we’ve written nothing down. Everything has been done on the calculator. This prevents copy errors and round-off errors.

What should a student show on his or her AP Exam? They are required to show what they are doing, or more precisely what they’ve asked their calculator to do. They need to write the equation they are solving with the solution next to it, but no intermediate work. They should indicate what A and C are. So

\ln (x)=x+2,\ x=0.158594\text{ and }x=3.146193

a=0.158594\text{ and }c=3.146193

Then show the integral and answer. Here again they may use f and g given in the stem or the actual expressions. There is no requirement that answers must be given to three decimal places, so there is no need to round.

\displaystyle \int_{a}^{c}{f\left( x \right)-g\left( x \right)dx}=1.94909

The next post in this series will show you a way to introduce the idea odf the derivative as the slope of the tangent line.

Back to School – 2016

Posted on by
1

aug 2016BACK TO SCHOOL – The three words we love and we hate.

I’ve been touching up the blog a bit this week. I hope the changes will make it easier to find posts on the topics you are interested in, and the blog more useful in general.

The THRU THE YEAR tab on the navigation bar at the top of the page has been updated. Links to my videos are now included. The monthly listings are organized so that you can stay ahead of your syllabus; to give you time to incorporate a new idea you like. So if your school starts in September, the August posts are where to start. The order of topics is not a day-to-day listing for you to follow or not even the order you must teach them in. So feel free to look around and rearrange.

The latest post appears at the top of the page. Below that are the four FEATURED POSTS. I will keep the current group in place for a while. These four posts concern the new Course and Exam Description (CED) for the AP Calculus program. The AP Calculus Concept Outline and the Mathematical Practices will be a help you understand the AP courses. The Getting Organized post will show you a way to organize your course using a Trello board that is already started for you.

Below the Featured Posts are the latest posts, newest on top. At the end of each post is a comment button so you can add your comments, suggestions, and ideas. Please use it.

You can find topics by using the SEARCH box on the right below the featured posts. Below that is a POST BY TOPIC drop-down list and a chronological ARCHIVES drop-down list.

If you cannot find what you need please let me know and I’ll see what I can do to help. I really could use some suggests for posts. My email is lnmcmullin@aol.com.

My plan for the coming months is to write posts on simple graphing calculator use for AP Calculus – ideas that I hope you can use to help your students understand what’s going on better. Look for them.

I hope the blog will make your year a little easier.

Have a good one!